If dark matter is fuzzy, then how fuzzy is it? - A gravitational lens has the answer
Dark matter, which makes up over 80% of the mass in the Universe, does not absorb or emit light, interacting with light and normal (baryonic) matter only through its gravitational pull. The nature of dark matter is one of the major open questions in astrophysics and cosmology. One theoretical model for dark matter, known as fuzzy dark matter (FDM), is predicted to leave a very specific imprint on light that is bent around a massive galaxy in a phenomenon called gravitational lensing. By examining the radio light in a gravitational lens system observed at extremely high angular resolution, we have determined just how “fuzzy” the dark matter can be.
In the quest to discover the nature of dark matter, many different theoretical models have been proposed. Most of these involve a new type of fundamental particle that interacts with other matter only through gravity. Particles with different masses are predicted to influence the small-scale distribution of the dark matter on galactic scales. For instance, cold dark matter (CDM) with a very massive particle would produce many compact, relatively low-mass clumps of dark matter orbiting every galaxy. In the opposite extreme, an ultra-light dark matter particle (ULDM), commonly called fuzzy dark matter (FDM), is predicted to produce a very blobby, wavy distribution of dark matter in every galaxy.
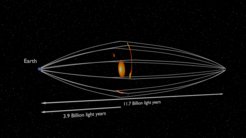
Gravitational lensing is one of the most promising ways to probe the nature of dark matter. As light from a distant source is bent around a lens galaxy in-between, we see several magnified and distorted copies of the source image (see the figure 1). The small-scale distribution of dark matter, which can be thought of as a “texture” in the gravitational lens, imprints a subtle signal on the lensed images that can be observed, if the observation is sensitive enough. In a lens galaxy containing fuzzy dark matter, this effect is similar to a pane of glass with a wavy pattern molded into it – the kind that is used in bathroom windows. It allows light to pass through, but distorts the image so that we cannot clearly see what is on the other side. By looking for the presence (or absence) of such subtle wavy distortions in the light passing through a gravitational lens, we can infer the mass of the dark matter particle. In fuzzy dark matter theories, a lighter particle produces a “fuzzier” lens, so we can use a gravitational lens observation to place a lower bound on the particle mass.
Our ability to see this fuzzy texture in the density of gravitational lens galaxies is limited by the angular resolution of the observation, or equivalently the size of the smallest features we can make out on the sky. Therefore, we get the best sensitivity to the dark matter particle mass by observing a gravitational lens system at the highest angular resolution possible. We achieve this using very long baseline interferometry (VLBI), a radio astronomy technique that combines signals from radio antennas all across the Earth. For this work, we used a VLBI observation of the gravitationally lensed radio jet MG J0751+2716 with an angular resolution of just 5 milli-arcseconds, or roughly one millionth of a degree. In the VLBI images, we can see the long, thin gravitational arcs that are perfect for searching for the small-scale gravitational perturbations that would be imprinted by fuzzy dark matter.
So, if dark matter is fuzzy, then just how fuzzy is it?
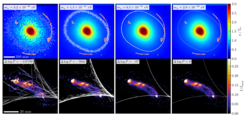
We generate many thousands of simulated fuzzy gravitational lenses with different dark matter particle masses (see figure 2). For each of these fuzzy lens models, we compute the likelihood that that such a lens could have produced the observed data. By computing thousands of these likelihoods over a range of proposed particle masses, we infer the probability that the dark matter consists of a particle of a particular mass, given the observed data. If the lens model is too fuzzy, the reconstructed source image becomes physically unrealistic. As the particle mass increases, we reach a point where the data cannot distinguish a fuzzy lens from a perfectly smooth lens model. We find that with 95% certainty, a mass of a fuzzy dark matter particle is not lower than of 4∙10-21 eV.
This work demonstrates the power of high-resolution VLBI observations to probe the nature of dark matter using strong gravitational lenses. Fuzzy dark matter is just one of many different theoretical models for dark matter. In future work we will use VLBI observations of gravitational lenses to constrain other dark matter models, including warm dark matter (WDM) and self-interacting dark matter (SIDM).













