Tsunamis and Ripples: Effects of Scalar Waves on Screening in the Milky Way
General Relativity has been great at reproducing and predicting phenomena in the Solar System, such as the bending of light by the Sun. However, observational data from supernovae suggest that the expansion of the universe is accelerating and this only be explained in the theory with an additional element. General relativity with a cosmological constant (the so-called ΛCDM-model) is consistent with all current observations - but is plagued by the infamous cosmological constant problem, i.e. nobody can explain the physical nature of this constant.
An alternative is to consider a theory of gravity that reduces to general relativity in the solar system but works differently on cosmological scales, such that it naturally drives the acceleration. In our analysis, we introduce a scalar field that interacts with matter, giving rise to a fifth force that makes matter deviate from their geodesics. Furthermore, the interaction depends on the composition of a massive body, thus allowing for environment-dependent behaviour.
We can use this environment-dependent, specifically local matter density to allow for deviations from general relativity on large scales while hiding troublesome modifications in the solar system. This is called screening. Two examples of screening mechanisms are the symmetron and the chameleon mechanisms. The symmetron mechanism works by suppressing the coupling of the field to matter in high density regions, i.e. suppressing their interaction; while the chameleon field's effective mass increases somewhere dense, resulting in a very short-ranged fifth force, irrelevant to macroscopic dynamics.
Until recently, these screening mechanisms have been studied under the quasi-static approximation. This means that one takes the equation of motion and assumes that the scalar field evolves very slowly. We can then neglect time derivatives and obtain the familiar Poisson-type equation, just like in Newtonian gravity, which does not allow for wave propagation.
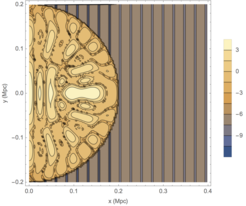
The relative effect of an incoming scalar wave on the deviation from general relativity in the Milky Way halo (technically: the parameterized post-Newtonian parameter γ.). The wave effectively travels through a medium with a refractive index different from one, similar to light passing through water.
In recent cosmological simulations, however, symmetron waves have been found when the quasi-static approximation is relaxed. Such scalar waves appear because the symmetron model allows for two distinct vacuum states in low-density regions. A small region of space can spontaneously flip its vacuum state to conform to its neighbour, this being more energetically favourable. Another source of scalar waves are violent astrophysical events, such as supernovae explosions, where less dense - hence unscreened - massive stars collapse into highly dense neutron stars or black holes, which are screened.
In the specific case of our solar system, the massive halo of the Milky Way should “screen” our neighbourhood, reducing the model to general relativity locally. However, it has been suggested that scalar waves of cosmological and astrophysical sources can observably disrupt this screening, such that a light-ray could experience an observably different amount of bending as it passes by the Sun.
As shown in other studies, an incoming spherical symmetron wave centred on the halo of the Milky Way can significantly disrupt the screening. These effects, however, could potentially violate the current observational bounds, hence ruling out previously viable models. Planar waves on the other hand are expected to be the more physically relevant wave configuration.
For astrophysical events with a source very far away from us, the planar-wave assumption should be very accurate. For waves of cosmological origin, which is only relevant to symmetron models here, the exact form of the incoming wave is less obvious as they are produced throughout the Universe and we are not necessarily in the far-field limit. Nevertheless, it is reasonable to expect that they can be represented as a superposition of plane waves with random wave vectors and phases.

This picture illustrates the refraction of light by a glass of water, which has a refractive index for visible light greater than one.
This caveat motivated us to gain a better physical understanding of this scenario. We quantitatively investigated the impact of scalar waves by solving the full field equation linearised in the wave amplitude. We find that inside the halo, the field becomes massive due to screening, which means that its phase velocity is modified. Analogous to light waves being refracted when they pass through water, the wavefronts are then bent.
We then studied the effect of the waves on the deviation from general relativity near the solar system's position within the halo. We find that planar incoming waves are significantly less disruptive than their spherical counterparts. This is of purely geometrical origin. Spherical waves focus a large amount of energy on the inner parts of the halo, where the solar system resides, while the more physical planar waves do not.
So while waves do propagate inside the halo in symmetron models and could potentially have consequences for models that are just marginally screened, the effects are much smaller than previously thought. Still, the effects of other factors, such as the halo’s density profile, and parameters of the model and incoming wave, are yet to be explored.













